The ‘willingness to pay’ (WTP) method was first applied in the health area in the famous study of WTP to avoid heart attacks, by Acton (1973). WTP for health is an issue in individual (personal) and societal (public) decision making about health care. The term usually refers to individuals’ willingness to spend money personally, i.e., ‘out of pocket,’ to obtain health gains for themselves or to avoid health losses or reduce health risks for themselves. WTP in this sense can be observed both in actual behavior and in responses to hypothetical questions. In either case, WTP is interpreted as an indicator of how much personal satisfaction or well-being (often called ‘utility’) individuals derive from (or believe they derive from) different health outcomes. It is thought that aggregate WTP out of pocket in a group of people benefiting from a program may be used as an indicator of how much money society should be willing to spend on the program, although this depends on the funding arrangements (whether health care is publicly or privately financed) and who is asked (patients or the public).
The satisfaction and well-being individuals derive from health outcomes may be measured in different ways. Most of these, including quality-adjusted life-years (QALYs) and disability adjusted life years (DALYs), aim at measuring the value of different health outcomes relative to each other with a view to aiding priority setting within given health budgets. The special property of measurements in terms of WTP is that they purport to establish the value of health outcomes relative not only to each other but also to other goods and services. In principle, WTP data may thus inform decisions not only about priority setting within given health budgets but also as to how much money should be allocated to health services versus other goods and services.
In the following, the authors’ focus is first on the meaning and measurement of individual WTP and the aggregation of this across individuals. Actual WTP for different technologies and programs in a public health service can be different from the simple individual aggregate, most notably because of various concerns for equal access and equity. The authors return to this important point in the Issues section at the end.
Welfare Theoretical Background And Application (In Principle) To Health Technology Assessment
What Is Willingness to Pay?
In standard welfare economics, maximum willingness to pay represents the theoretically correct measure of ‘strength of preference’ for, or value of, a commodity. In areas of public-sector activity, such as health care, in which conventional markets do not exist, decisions still have to be made about how best to use limited resources. This requires valuation of both resource costs of interventions and their benefits (the benefits being health gain and other sources of well-being). This can be elicited either in surveys by use of hypothetical WTP questions – essentially, the contingent valuation approach in which respondents state values for the good in question – or revealed as a result of observing real market-based choices. Owing to the rarity of the latter in health care, the contingent valuation method tends to be relied on more than revealed preference.
WTP focuses on the valuation of benefits, whereby a health care option may be described to a respondent and the person is asked what is his/her maximum WTP for it. In principle, with this type of information, the combination of interventions in cash-limited publicly funded systems could be chosen, which maximizes the value of benefits (possibly distributionally weighted) to the community. In privately funded systems, WTP information would more likely be used to assess whether aggregate WTP for a particular health care intervention exceeds costs; if it does, the implication is that this intervention can be added to the benefits package. In each of these cases, WTP is necessarily treated as a cardinal measure of value, i.e., a measure which captures strength as well as direction of preference.
It is important to distinguish WTP, as a measure of benefit, from the price of a good. In many cases, people would be willing to pay more than the market-clearing price of a good. For any individual, the difference between benefit, as represented by his/her maximum WTP for the good, and the price paid by him/her for the good represents a gain in well-being from having the good provided at the market price.
Willingness to Pay And Levels Of Decision Making
Willingness to pay methods have been used at three main levels of decision making in health care. The first level is that of patients within a clinical area being asked to choose between close substitutes of the sort that might be evaluated within a randomized trial. It could be argued that when making such choices between therapies, the people best placed to judge are patients who will receive the therapies. The second level is that where geographically defined health authorities have to prioritize across such clinical areas in a publicly funded system (e.g., as in one study, by Olsen and Donaldson (1998), comparing helicopters, hearts, and hips). Here, it could be argued that those best placed to decide are taxpayers (or those who paid taxes in the past). In more insurance-based systems, where the decision might be about whether to add a program to the benefits package, those same people might be asked about their WTP for that program alone, the decision being based on whether the aggregate WTP is greater than the costs of said program. The different scenarios that emanate from these contextual backgrounds, and the ways that WTP can be used within them, have been laid out by O’Brien and Gafni (1996) and Shackley and Donaldson (2000).
Internationally, the advent of Health Technology Assessment (HTA) agencies has now taken WTP methodology to the national level. Many of the recommendations made by HTA organizations involve considerations of the costs and benefits of single interventions, where there is no obvious basis for a comparison between alternatives, apart from with the costs and effects of treatments that constitute current practice or the status quo. In such cases, the decision about whether to provide the intervention is not obvious and hence gives rise to the question of what monetary value to place on a gain of one QALY.
All of the above scenarios also give rise to the question of whether WTP can be used to value wider benefits of health care (e.g., location of care and process utility) over which people have preferences but which might not be captured easily by narrower, health-focused methods, such as QALYs.
Measurement Of Individual Willingness to Pay And Estimation Of Aggregate Value
In 1963, the first empirical application of willingness to pay, published in a journal, was in the area of environmental policy evaluation. During the 1970s, the method was further developed in studies of the valuation of saving lives, as applied to safety and transport policies, largely through the work of Jones-Lee (1974) and Jones-Lee et al. (1985). This latter body of work is of great relevance to the issues faced currently in health as the development of methods to elicit a value of saving a life has strong parallels with the challenge of valuing a QALY (or ‘healthy year’). Therefore, in this section, the authors commence with an introduction to measurement methods by focusing on lessons learned in area of safety (especially the issue of how to estimate the value of a prevented fatality, or VPF), before moving to how these lessons have been built on in deriving estimates of the value of a QALY.
Revealed Preference Versus Contingent Valuation In Safety
Basically, the revealed preference approach involves the identification of situations in which people actually do trade-off income or wealth against physical risk – for example, in labor markets where riskier jobs can be expected to command clearly identifiable wage premia. By contrast, the contingent valuation approach applied to safety typically involves asking a representative sample of people more or less directly about their individual WTP for improved safety (or, sometimes, their willingness to accept compensation for increased risk).
The difficulty with the revealed preference approach when applied to labor market data is that it depends on being able to disentangle risk-related wage differentials from the many other factors that enter into the determination of wage rates. The approach also presupposes that workers are well informed about the risks that they actually face in the workplace. In addition, those whose jobs do carry clearly identifiable wage premia for risk may not be representative of the work force as a whole, in that such people almost certainly have a below-average degree of risk-aversion.
The great advantage of the contingent valuation approach is that it allows the researcher to go directly and unambiguously to the relevant wealth/risk trade-off – at least, in principle. However, the contingent valuation approach has the disadvantage of relying on the assumption that people are able to give considered, accurate, and unbiased answers to hypothetical questions about typically small changes in already very small risks.
Aggregation
So, under what has naturally come to be known as the ‘WTP’ approach to the valuation of safety, one first seeks to establish the maximum amounts that those affected would individually be willing to pay for (typically small) improvements in their own and others’ safety. These amounts are then simply aggregated across all individuals to arrive at an overall value for the safety improvement concerned (see example in following paragraph). The resultant figure is thus a clear reflection of what the safety improvement is ‘worth’ to the affected group, relative to the alternative ways in which each individual might have spent his or her limited income. Furthermore, defining values of safety in this way effectively ‘mimics’ the operation of market forces – in circumstances in which markets typically do not exist – insofar as such forces can be seen as vehicles for allowing individual preferences to interact with relative scarcities and production possibilities to determine the allocation of a society’s scarce resources.
To standardize values of safety that are derived from the WTP approach and render them comparable with values obtained under other approaches (such as gross output – see Jones-Lee (1994)), the concept of the prevention of a ‘statistical’ fatality or injury is applied. To illustrate this concept, suppose that a group of 100 000 people enjoy a safety improvement that reduces the probability of premature death during a forthcoming period by, on average, 1 in 100 000 for each and every member of the group. The expected number of fatalities within the group during the forthcoming period will thus be reduced by precisely one, and the safety improvement is therefore described as involving the prevention of one statistical fatality. Now suppose that individuals within this group are, on average, each willing to pay ₤w for the 1 in 100 000 reduction in the probability of death afforded by the safety improvement. Aggregate WTP will then be given by ₤w×100 000. This figure is naturally referred to as the WTP-based value of preventing one statistical fatality (VPF) or alternatively as the value of statistical life (VOSL). So, for instance, if w = ₤10, VOSL = ₤1 000 000 in our example.
Clearly, in the above example, average individual WTP, ₤w, for the average individual risk reduction of 1 in 100 000 is a reflection of the rate at which people in the group are willing to trade-off wealth against risk ‘at the margin,’ in the sense that the trade-offs typically involve small variations in wealth and small variations in risk. Empirical work on the valuation of safety thus tends to focus on these individual marginal wealth/ risk trade-off rates.
On a somewhat more cautionary note, it is extremely important to appreciate that, defined in this way, the VPF is not a ‘value (or price) of life’ in the sense of a sum that any given individual would accept in compensation for the certainty of his or her own death – for most of us, no finite sum would suffice for this purpose so that in this sense life is literally priceless. Rather, the VPF is an aggregate WTP for typically very small reductions in individual risk of death (which, realistically, is what most safety improvements really offer at the individual level).
In valuing safety it is also useful to have a valuation of an averted statistical injury (VSI). The VSI is generally pegged against the VOSL rather than estimating WTP for an injury. To do this, a standard gamble exercise can be used to calculate the marginal rate of substitution (MRS) of an injury against death. The value of preventing this injury is then the fraction of the VOSL that is given by the MRS, i.e., if the MRS is 0.09, the value of preventing this injury will be 9% of the VOSL.
Recent Developments In Valuing Life
Viewed from an historical perspective, both quantitative and qualitative research have tended to cast doubt on the reliability and validity of WTP values for safety derived through the direct contingent valuation method. As well as sequencing and framing effects, a prominent issue has been the lack of ability of the method to account for embedding and scope. That is, respondents tend to view safety improvements as a ‘good thing’ and, therefore, will often state much the same WTP for different sizes of risk reduction, whether for fatal or nonfatal injuries. It may be unreasonable to expect respondents to give accurate answers to hypothetical questions, which involve direct trade-offs between wealth and small reductions in risk.
In view of these difficulties with the direct contingent valuation approach, Carthy et al. (1999) suggested a lessdirect, ‘chained’ approach, which breaks down the valuation process into a series of more manageable steps. More specifically, respondents are first presented with a question asking them about their WTP for the certainty of a complete cure for a given nonfatal road injury and their willingness to accept compensation (WTA) for the certainty of remaining in the impaired health state. On the basis of reasonable assumptions concerning underlying preferences, it is then possible to obtain an estimate of each respondent’s marginal rate of substitution of wealth for the risk of the nonfatal injury as a weighted average of his/her WTP and WTA responses. Respondents are then presented with a ‘standard gamble’ (SG) question aimed at determining the ratio of individual marginal rates of substitution of wealth for risk of death relative to the corresponding marginal rate of substitution for risk of the nonfatal injury. The monetary value from the first stage can then be combined with the ratio from the second stage to obtain a WTP for reduced risk of death.
The approach is, perhaps, more realistic in that most people can relate to giving a monetary value for avoiding a nonfatal injury of the sort they are likely to have experienced, and people are not asked directly to place a monetary value on a small risk reduction. The method has shown promise in terms of being subject to less marked embedding effects and other biases than earlier approaches.
Linking Safety And Health: Modeling The Value Of A QALY
As noted, the most common measure of benefits applied in health economic evaluation is the QALY. Although the cost per QALY gained for technology ‘A’ can be compared with the cost per QALY gained of technology ‘B’ (and hence the relative efficiency of A vs. B can be assessed), weighing up costs (measured in monetary terms) and health benefits (measured in QALYs) for a single technology is problematic. As a result, health care decision makers have tended to invoke decision rules (such as cost per QALY limits above which technologies are unlikely to be recommended for adoption). To generate research evidence to underpin such decision rules, there has been international interest in the estimation of the monetary value of a QALY. If such a value could be estimated, then costs and benefits could be compared using the same metric (i.e., money), and one could more readily judge whether benefits offset costs. Existing evidence from the economics of safety literature is a natural starting point for such a project.
A straightforward way to combine work on health and safety valuation is to take the well-established VOSL based on research into road safety and, from it, attempt to model the monetary value of an year of life. This can reasonably be interpreted as the value of a healthy year, which is the same as a QALY. Indeed, this is what was attempted in the UK Social Value of a QALY (SVQ) project and more recently in the European Value of a QALY project (EuroVaQ). A simplified version of the method of transforming the VPF into a value of a QALY is presented in Box 1. However, the value resulting from this would reflect a particular QALY type. By QALY type, the authors mean that QALYs can be generated in at least two ways, these being by adding years to life expectancy or by enhancing the quality of remaining life years without extending life. The former can be further subdivided into avoiding immediate threats to life – hereafter called ‘life saving,’ or adding years to the end of one’s life – hereafter called ‘life extension.’ The procedure outlined in Box 1 reflects the first of these, although a more-sophisticated approach, still using the VOSL as a basis, was also employed to model the value of a QALY arising from life extension as opposed to life saving.

Valuing Improvements In Quality Of Life
Willingness to pay procedures have been used directly by the UK Department for Transport to derive the value of preventing a serious injury (VSI). From such data, the monetary value of health gains (as measured by instruments in the QALY field) can be estimated. But one can also combine a monetary value for a health gain with health state utility scores to achieve values for different improvements in quality of life. The simplest way to do this is to use established utility tariffs in instruments such as the EQ-5D, the Health Utilities Index, the 15-D, etc. For instance, if a survey respondent was willing to pay a maximum of ₤3000 WTP for a move from a more-impaired health state for 1 year to a less-impaired state for the same period, where the tariff difference between the states is 0.1, then the WTP for a QALY implied by this would be ₤30 000 (i.e., ₤3000/0.1) for that individual. If repeated over several respondents and scenarios, a representative WTP for a QALY could be derived (Gyrd-Hansen, 2003).
Others have combined WTP questions and utility scores in the same survey, for example, in the social value of a QALY studies in the UK and Spain, the feasibility of doing this was assessed by presenting members of the public with appropriately framed valuation questions in a survey – see Box 2 for example health states, and Box 3 for example question to illustrate how changes in quality of life and WTP were estimated and combined (each from the UK study).

From Box 3, it can be seen that any individual respondent would be faced with a set of WTP and standard gamble questions, the two sets then being combined in different ways to arrive at values of a QALY. The reason for having respondents undertake a health state utility assessment exercise, rather than combine WTP values with a preexisting tariff (such as that which exists for the EQ-5D quality-of-life system), was that the researchers wanted each respondent’s WTP value to be combined with their own personal health state utility value for purposes of internal consistency.

Examples Of Applications In Safety And Health
Values Of Life
Turning to the question of the figures that are actually applied in practice, WTP-based values of safety are currently used in road project appraisal in the UK, USA, Canada, Sweden, and New Zealand, with several other countries employing values that have been substantially influenced by the results of WTP studies. More specifically, in the UK, the Department for Transport currently employs a figure of ₤1.64 million in June 2007 prices for the prevention of a statistical fatality in its roads project appraisal. In turn, the Department’s values for the prevention of serious and slight, nonfatal injuries are ₤185 220 and ₤14 280, respectively, again in 2007 prices.
In the USA, the US Department of Transportation currently values the prevention of a statistical road fatality at US$6 m (approximately ₤3.96 m). In turn, Transport Canada applies a WTP-based value for the prevention of a statistical fatality of Cdn$ 4 m (approximately ₤3.22 m) based on a survey of the literature. The WTP values used in Sweden and New Zealand were derived under the contingent valuation approach and in 2010 prices are SEK 18.5 million (approximately ₤1.8 m) and NZ$ 3.56 million (approximately ₤1.7 m).
WTP For A QALY: Estimates From Modeling Studies
Table 1 gives a typical set of values of a QALY that have arisen from the UK-based modeling study described above (see Box 1). It would seem that different ‘QALY types’ would imply different values. Based on WTP for life saving (to reduce the risks of life-threatening events), values close to ₤70 000 per QALY were produced, as compared to values approximately ₤35 000 for a life-extending QALY (at the end of life). Estimating gains from improvements in quality of life, with no increase in number of remaining years, produced a lower value of approximately ₤10 000 per QALY. These differences are striking, and further studies are needed to clarify them.
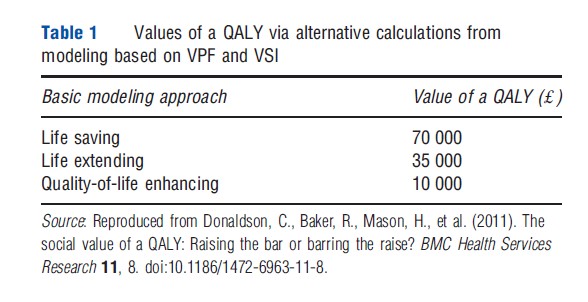
The issue of willingness to pay for QALY types has been explored recently in subsequent surveys on the ‘European value of a QALY.’ It is also worth noting that the VPF itself is just over nine times the value of preventing a statistical injury. That there is no single value of a QALY is in line with other published views, the lowest value also being reflective of earlier published studies, which looked at the value of QALY gains arising from quality-of-life enhancement only.
A small number of other studies have modeled the value of a QALY from a Value of a Statistical Life. Using Australian data Abelson (2003) reported a value of AUS$108 000 (approximately ₤59 000). In Sweden, a value of US$90 000 (approximately ₤64 000) was reported by Johannesson and Meltzer (1998), whereas Hirth et al. (2000) calculated a value of US$161 305 (approximately d115 000).
Values Of A QALY From Survey Research
Because the surveys referred to above from the UK and Spain (see Baker et al. and Pinto Prades et al. in further reading list) were intended only as feasibility studies, they were based on small samples not necessarily representative of the population as a whole. Nevertheless, the work suggests that it is feasible to conduct a survey to elicit monetary values for a QALY from a representative sample of the public so long as the procedure is broken down into manageable steps. However, it also became apparent that the mean estimates produced by such questions are particularly prone to the influence of ‘outlier responses,’ and that great care is therefore required in the selection of centraltendency measures. The most common example of an outlier was that many people were willing to take only very small risks of a more adverse outcome to avoid the stomach and head health states in the standard gamble questions or were even not willing to gamble at all. As well as such ‘floor’ effects respondents may also have a WTP ceiling (or budget constraint), an amount they express whether for a small or large perceived gain. Thus, when WTP values and health state utilities are combined in such circumstances, the implied WTP per QALY for such individuals can be so high as to lead to an implausible population average WTP per QALY across the whole sample. This was indeed the case in these studies, with the value running into several millions of pounds/euros! Other ways of managing the data can be used, however. For example, if mean WTP and mean QALY loss are combined (a ‘ratio of means’ as opposed to ‘mean of ratios’ approach), much more conservative values of a QALY emerge.
Issues
Willingness to Pay And Ability To Pay
On the face of it, it would seem that it is problematic to use willingness to pay measures to inform decisions about the allocation of resources for commodities, such as health care, for which such allocation is supposed to be on the basis of (some notion of) need. This is because WTP is obviously associated with ability to pay. However, Government departments in the UK and in other countries employ WTP-based values for the prevention of a statistical premature fatality (VPF) that are based on central-tendency measures (typically arithmetic means) of the population distribution of individual WTP for risk reduction. The fact that these VPFs are then applied uniformly to all groups in society whatever the income levels of members of the group clearly entails the implicit use of inverse-income distributional weights and further overcomes the challenge of ability to pay influencing WTP.
It would also appear that there is already a precedent in public-sector decision making for (at least implicitly) applying distributional weights to individual WTP for reductions in risks to life in order to arrive at an overall value in the form of a population mean (or possibly median) of individual values. This represents the state of the art of dealing with any such biases.
Validity And Scope
A major focus in the environmental willingness to pay literature with respect to validity has been on scope effects: especially whether respondents are willing to pay more for greater amounts of the good being valued than for smaller ones, as one would expect. Carson (1997) showed that most studies (31 of 35 reviewed) reveal sensitivity to scope. Despite such positive results, doubts still remain about WTP values elicited through hypothetical surveys, and scope tests have taken on the status of being the ‘acid test’ for any particular study. As in other areas of application, results in health have been mixed (Smith, 2001; Olsen et al., 2004). Also, this has led to more qualitative methods being used to examine the thought processes underlying respondents’ stated values, a trend which will likely (and justifiably) continue.
Valuing Others
So far only passing reference has been made to people’s concern – and hence willingness to pay – for others’, as well as their own health and safety. Insofar as people do display such ‘altruistic’ concern, then one would naturally expect that it would be appropriate to augment the WTP-based VPF to reflect the amounts that people would be willing to pay for an improvement in others’ safety. However, it turns out that under plausible assumptions about the nature of people’s altruistic concern for others’ safety on the one hand and their material well-being on the other (the latter being reflected by their wealth or consumption), augmenting the VPF to reflect WTP for others’ safety would involve a form of double counting and would therefore ultimately be unjustified. For example, suppose that individual A is concerned not only about individual B’s safety but also about the latter’s wealth or consumption. Furthermore, suppose that individual A’s altruistic concern for B is ‘pure,’ in the sense that it respects B’s preferences. Although A will then regard a reduction in B’s risk of premature death as a ‘good thing,’ he/she will also regard the increase in B’s taxation (or other expenditure) required to finance the risk reduction as an exactly offsetting ‘bad thing.’ Taking account only of A’s WTP for B’s safety improvement would therefore quite literally involve double counting. Thus, the issue of whether and how people’s concern for others’ safety ought to be taken into account under the WTP approach hinges on the essentially empirical question of the relationship between such concern and concern for others’ wealth or consumption. Detailed discussions of the issue of altruism and safety exist in the literature, and it would seem that similar arguments apply to societal WTP values elicited in the health field.
Actual WTP In A National Health Service With Concerns For Equity
As noted above in the Section on Valuing Improvements in Quality of Life, willingness to pay for improvements in quality of life can be estimated by combining WTP with individual utility scores for health states (measured on the 0–1 scale used in QALY calculations). For instance, if a national health service’s WTP for a gained healthy year is ₤30 000, the WTP for improving a person’s health (utility) by 0.1 for 1 year may be estimated to be 0.1×30 000 = ₤3000. But this presupposes that individual utilities for health states correctly reflect societal pReferences: for priority setting – and hence correctly indicate societal WTP. There is much evidence that this assumption does not hold in societal decisions about resource allocation among groups of patients with different degrees of severity of disease and among groups with equal interests in treatment but different capacities to benefit from treatment. Most notably, in several jurisdictions the general public and societal decision makers have been shown to value utility gains more the more severe the initial condition is (Nord, 1999; Shah, 2009). To use conventional utilities from the QALY field to estimate what would be reasonable for a national health service to be willing to pay for technologies and programs that improve quality of life is thus problematic.
To capture concerns for equity, Nord (1999) suggested a type of priority weights for life years with a structure that is different from that of utilities normally used in QALY calculations. In principle, such weights can be used instead of conventional utilities when combining with a public health service’s WTP for gaining a healthy year to estimate WTP for improvements in quality of life. This is an area of continuing research. There is at present no universal agreement as to what would be the right alternative weights to use for this purpose.
A similar point about equity can be made regarding WTP for gained life years. Even if a national health service indicates a WTP of, for instance, ₤30 000 for one gained healthy year (one QALY), it does not follow that N gained years should give a WTP of N × 30 000. A national health service may for instance consider that this would lead to unjustified age discrimination in priority setting in surgery and other one-time treatments of life-threatening conditions. It does not follow that a gained life year in a state of reduced health, say at utility level 0.8, should give a WTP of only 0.8 × 30 000 = ₤24 000, as this by many would be regarded as unfair discrimination against people with chronic disease or disability.
Conclusions
Measurements of individual willingness to pay for health benefits are potentially useful to examine whether the individual welfare gains from health programs outweigh their opportunity cost, i.e., outweigh the welfare gains that could have been obtained by spending the resources on other goods and services instead. In this, the WTP approach has an advantage over approaches that use QALYs or DALYs. As with other approaches to valuation of health care, there are various methodological challenges associated both with measurement and aggregation of WTP. There is also an important distinction between estimating aggregate individual WTP and determining what should be the WTP in a national health service that strives for both efficiency and equity in health care. Further research on WTP measurements will help to clarify the potential and the role of the WTP approach in health care resource allocation.
References:
- Abelson, P. (2003). The value of life and health for public policy. The Economic Record 79, S2–S13.
- Acton, J. P. (1973) Evaluating Public Programs to Save Lives: The Case of Heart Attacks. Report No. R950RC, Santa Monica: RAND Corporation.
- Carson, R. T. (1997). Contingent valuation surveys and tests of insensitivity to scope. In Kopp, R. J., Pemmerhene, W. and Schwartz, N. (eds.) Determining the value of non-marketed goods: Economic, psychological and policy relevant aspects of contingent valuation methods. Boston: Kluwer.
- Carthy, T., Chilton, S. M., Covey, J., et al. (1999). On the contingent valuation of safety and the safety of contingent valuation: Part 2 – the CV/SG ‘chained’ approach. Journal of Risk and Uncertainty 17, 187–213.
- Gyrd-Hansen, D. (2003). Willingness to pay for a QALY. Health Economics 12, 1049–1060.
- Hirth, R. A., Chernew, M. E., Miller, E., Fendrick, M. and Weissert, W. G. (2000). Willingness to pay for a quality-adjusted life year: in search of a standard. Medical Decision Making 20, 332–342.
- Jones-Lee, M. W. (1974). The value of changes in the probability of death or injury. Journal of Political Economy 82, 835–849.
- Jones-Lee, M. W. (1994). Safety and the saving of life: The economics of safety and physical risk. In Layard, R. and Glaister, S. (eds.) Cost benefit analysis. Cambridge: Cambridge University Press.
- Jones-Lee, M. W., Hammerton, M. and Philips, P. R. (1985). The value of safety: Results of a national sample survey. Economic Journal 95, 49–72.
- Nord, E. (1999). Cost-value analysis in health care. Cambridge: Cambridge University Press.
- O’Brien, B. J. and Gafni, A. (1996). When do the ‘dollars’ make sense. Towards a conceptual framework for contingent valuation studies in health care. Medical Decision Making 16, 288–299.
- Olsen, J. A. and Donaldson, C. (1998). Helicopters, hearts and hips: Using willingness to pay to set priorities for public sector health care programmes. Social Science and Medicine 46, 1–12.
- Olsen, J. A., Donaldson, C. and Periera, J. (2004). The insensitivity of ‘willingness to pay’ to the size of the good: New evidence for health care. Journal of Economic Psychology 25, 445–460.
- Shackley, P. and Donaldson, C. (2000). Willingness to pay for publicly financed health care: How should we use the numbers? Applied Economics 32, 2015–2021.
- Shah, K. K. (2009). Severity of illness and priority setting in healthcare: A review of the literature. Health Policy 93, 77–84.
- Smith, R. (2001). The relative sensitivity of willingness-to-pay and time trade-off to changes in health status: An empirical investigation. Health Economics 10, 487–497.
Further Reading:
- Baker, R., Bateman, I., Donaldson, C., et al. (2010). Weighting and valuing quality-adjusted life-years using stated preference methods: Preliminary results from the social value of a QALY project. Health Technology Assessment 14, 27.
- Donaldson, C. (1999). Valuing the benefits of publicly-provided health care: Does ‘ability to pay’ preclude the use of ‘willingness to pay’? Social Science and Medicine 49, 551–563.
- Donaldson, C., Birch, S. and Gafni, A. (2002). The pervasiveness of the ‘distribution problem’ in economic evaluation in health care. Health Economics 11, 55–70.
- Johannesson, M. (1995). The relationship between cost effectiveness analysis and cost benefit analysis. Social Science and Medicine 41, 483–489.
- Mason, H., Jones-Lee, M. W. and Donaldson, C. (2009). Modelling the monetary value of a QALY: A new approach based on UK data. Health Economics 18, 933–950.
- Pinto Prades, J. L., Loomes, G. and Brey, R. (2009). Trying to estimate a monetary value of a QALY. Journal of Health Economics 28, 553–562.
- Viscusi, W. K. (1978). Labor market valuations of life and limb: Empirical evidence and policy implications. Public Policy 26, 359–386.